Learning objectives: Introduction to Rotational Dynamics beginning with the symbols used and rotational kinematic equations. We then stepped into Torque, defined.

New Assignments: 

• Feel the Torque! This lab had students placing weights at various points on a meter stick and then holding the stick at predefined angles in order to calculate and feel the torque.
• Determination of Max Omega! This lab had students sitting on the “Sit and Spin” chair and simply measuring the maximum rotational velocity in RPM and Rad/sec.

The opening discussion was focused on the symbols used in Rotational Dynamics (defined in chapters 10.1 and 10.2 of the OpenStax text book. Of special interest was the comparison between linear kinematic equations an rotational kinematic equations (illustrated in on page 425). On the second day, we delved into Torque as a topic (described in chapter 9.2 in the text) first introducing the concept of a force being applied at a distance away from an axis of rotation, then doing a mini-lab “Feel the Torque” and finally walking over to the autoshop.. so students could use a Torque wrench to tighten a lug nut on an old truck to a pre-defined Torque specification. (we also watched some youtube clips of wheel standing drag racers) 

Learning Objectives

 

 

New Assignments: 

• Feel the Moment (of Inertia). Students considered the acceleration of an ordinary hammer about three different axis. For each axis, students were to consider the hammer (the handle and the ‘head’) as the Sum of geometric objects, each with its own, Moment of Inertia. The students are to both draw the shapes individually, and showing what the equation of Moment of Inertia is for each, and then show how the total Moment of Inertia is the ‘sum of the parts’.
• Video: Johannes Kepler  While Clark was out, students were to watch and take notes this short (30 min.) documentary on Kepler’s life and accomplishments. Students should focus their attention on how his upbringing, how he survived, the role that Tycho Brahe played in his life and his theories of planetary motion. What are the three Laws of motion which he finally determined?
• Homework set: Problems 1, 5, 7, 8 on page 275 and problems 3, 7, 8, 11 on pages 460-461 of the on-line text book.

This week introduced students to the concepts of Inertia for rotating systems. As in other systems, Inertia is the measurement of an objects ‘resistance to a change in velocity’. For objects travelling in a straight line, the measurement is simply the mass of the object measured in Kilograms. For a rotating system, the mass matters as well, but more importantly, the distribution of mass matters more. To begin the discussion, Clark considered a hammer, in which the head of the hammer (with significant mass) is rotated about a distant ‘axis of rotation’ and derived the fundamental equation for Moment of Inertia. From there we determined that for any distributed mass system, the total Inertia is simply ‘the ‘sum of the parts’. From here we considered various tables which present to us, the moments of Inertia for various shapes being rotated about various axis.

Learning Objectives

 

 

New Assignments: 

• Model Moment of Inertia lab. This lab had students attempting to model the moment of inertia of a system consisting of an aluminum plate with three masses located asymetricaly on top (one of which was a force/accelerometer). They applied a known torque to the system, measured the subsequent rotational acceleration and from that, deduced what the ‘measured’ Moment of Inertia must be for the system.
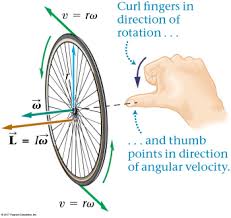
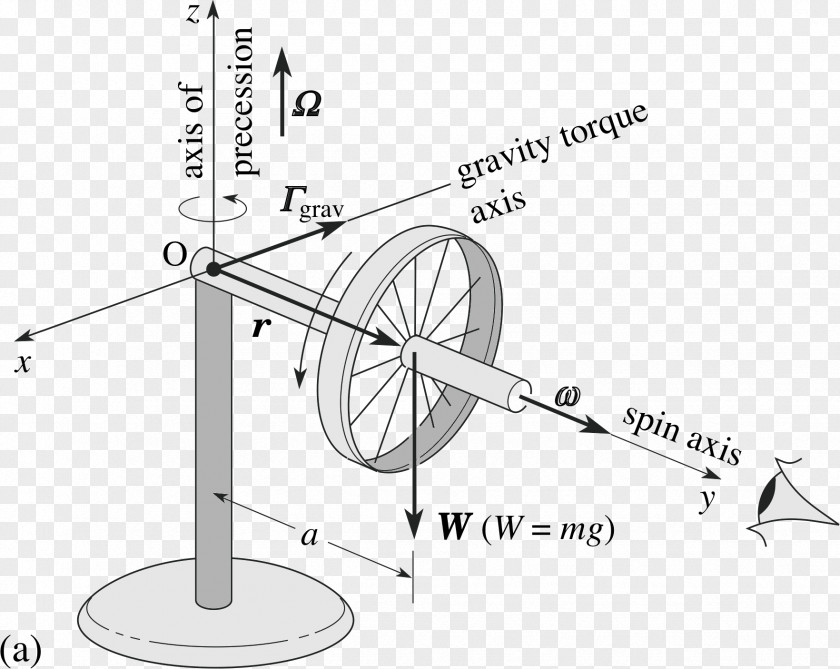
• rewrite: Why doesn’t the spinning wheel fall? Students were to ‘give back’ the lecture and demonstrations that Clark gave showing how ‘vector math’ predicts the solution of the wheel precessing about a new center-line. This Wikipedia page describes in detail, the torques which lead to precession.

This week introduced vectors as a way to describe various rotational dynamic properties such as angular momentum, torque and rotational velocity. The example given was the gyroscope effect of a spinning bike wheel not falling but instead preccess ing about an axis when released. Vector math is really the only way to consider this phenomena.

Learning Objectives

 

 

New Assignments: 

• Podcast: The Science of Friction. Students were to take a page of notes, highlighting the factors that affect friction, how we measure it, etc.
• On-line Practice Ap Test. Students should see how well they do in this test and we’ll discuss next week. (note: The students need to log into the AP Classroom website to take the test. They MAY work together to discuss).

This week began with continuing the discussion of the ‘inertia’ of a rotating system. The geometry of the mass matters more than the amount of mass (measured in Kgs).

As an exciting example of the Universe at Work.. we explored the world of Pulsars, Neutron Stars and Magnetars and how Conservation fo Angular Momentum is conserved and leads to objects  which spin at hundreds of times per second, but contain the mass of hundreds of Suns

With that in mind, the concept of Angular momentum and the conservation thereof, was introduced. For demonstration, Clark brought out a rotating platform/stool, and demonstrated how holding a spinning bike-wheel in different orientations resulted in the ‘system as a whole’ responding. Also, introduced, was the concept of using vectors to represent roational values (omega as the rotational velocity and L as the momentum vector.. L = I omega).

On Thursday, we stepped into the demonstration of the wheel being spun up and then being suspended by the end of axel rod. Clark then walked through the vector physics predicting the observed motion centered on the idea that ‘if you can see the direction of the forces/torque, you can also see the direction fo the change in velocity/omega). Students were tasked with taking notes during the lecture and then rewriting them such that they look like a college text book section on the matter.

• Introduction of Kinetic Energy of a rotating system and how we can use conservation of energy principals to rotating systems. Specifically we considered a disk rolling down a ramp and how the gravitational potential energy would be distributed between the rotational kinetic energy and the linear (translational) kinetic energy.
• A special emphasis was placed on how to link linear motion (of a bucket falling) to rotational motion (of the pulley supporting the bucket).

 

 

New Assignments: 

• Text book problems (page 461) 13, 15, 20, 22, 27, 32, 39.
• chpt 10_rotational dynamics and energy

This week students were introduced to concepts of conservation of energy in rotating systems. Just like in linear velocity systems, work is defined as force times distance, but in this case, we consider the force acting as a torque causing a system to rotate through an angular displacement. And how kinetic energy of a rotating system is analagous to kinetic energy in a linear system (with KE = 1/2 I Omega squared.).

The main ‘new’ discussion was examining a problem in which a bucket suspended from a rope wrapped around a pully will descend by using the transformation from tangential velocity to rotational velocity to ‘tie’ the two components of the problem together.. Also discussed, was how we can use concepts of energy to determine the rotational velocity and linear velocity of a disk rolling down an incline plane.

 

 

New Assignments: 

Learning Objectives for the week.

• A quick review of Power = work/time (joules/sec = Watts or Horse Power)
• Nelson Racing Engines main website
• NRE 2500 HP dyno test
• GM Performance Parts Crate Engines page (632 c.. = 1000 HP!)

New Assignments: 

• Test 1:(Spring) Rotational Dynamics
• Particle Fever (video) Students took a page of notes on the development of the Large Hadron Collide

Learning Objectives for the week.

New Assignments: 

• Article: Probing the Proton. Students annotated this article. (A good follow up to the video: Particle Fever).

A high speed introduction to Simple Harmonic Motion.

Learning Objectives for the week

• Restoring forces
• Spring Constant
• Conservation of Energy in an oscillating system

New Assignments: 

• oscillating systems. This lab made use of Smart Cars on incl

This week focused on how we can measure the various forces and dynamics of oscillating systems. Of central interest was the summation of vectors leading resulting in restoring forces and how by writing functions within our Excel or Google Sheets data sets, we can follow the energy of the system as it oscillates back and forth (the kinetic energy of the car, the gravitational potential and the Spring potential energy must all add to a ‘fixed number'”. Real systems however, dissipate energy and the system is ‘damped’ by the slow recovery of the rubber bands.. (so the total energy should slowly decrease asymptotically down to a lower value than we started with).

Also discussed was the list of topics students should be familiar with for Friday’s practice AP Test on Rotational Dynamics. Students were encouraged to review Unit-7-AP-Physics-1

Learning Objectives for the week

• Putting theory to practice; namely taking the ‘text book’ problem of a mass and spring system, we set up our own to see for ourselves.

New Assignments: 

• Share a problem. The idea with this activity, is that each day, students will take turns sharing with the class, a particular physics problem which at first stumped them and then they figured out. Clark will maintain a calendar of students presentation dates.

This week focused on how we can measure the various forces and dynamics of oscillating systems. Of central interest was the summation of vectors leading resulting in restoring forces and how by writing functions within our Excel or Google Sheets data sets, we can follow the energy of the system as it oscillates back and forth (the kinetic energy of the car, the gravitational potential and the Spring potential energy must all add to a ‘fixed number'”. Real systems however, dissipate energy and the system is ‘damped’ by the slow recovery of the rubber bands.. (so the total energy should slowly decrease asymptotically down to a lower value than we started with).

Also discussed was the list of topics students should be familiar with for Friday’s practice AP Test on Rotational Dynamics. Students were encouraged to review Unit-7-AP-Physics-1

Thursday’s class centered on Clark’s own lab experiment (oscillating systems) and the conversation of how each energy system requires its own version of the position data. (ie., to calculate energy stored in the spring, the position data set places the zero mark at the top whereas for calculations of gravitational potential energy, the zero mark needs to be at the bottom).

Learning Objectives

• Wave speed is ‘fixed’ (for a given substance)
• Waves can pass through each other without interacting
• Waves can ‘interfere’ with each other in the substance
• Waves can reflect
• Introduction to harmonics (the series of frequencies and wave forms which can exist in a system).

New Assignments:

• slinky waves_spring 25
• Waves on a string simulator. (student handout: waves on a string)

This week began with an introduction to waves. Waves is the natural extension of oscillating systems, in which particles are loosely connected (with the degree of attachment dependent on ‘the system’). For all waves, we model the medium as a series of masses connected by a series of springs.. therefor the two factors that determine the speed that waves pass through the medium are a) the inertia of the particles and b) the ‘stiffness’ (or Spring constant) of the connections between particles. A key point of interest, is that a wave is a transfer of energy through the system, NOT a transfer of mass (the mass stays essentially ‘in place’, only oscillating back and forth around a fixed point of equilibrium).

Friday students spent time on the practice AP test for oscillations.

Learning Objectives

• Considering how wave harmonics can exist in air (sound).. and using fixed frequency tuning forks to determine the speed of sound
• Predicting wave forms in a cylinder ‘open at one end’
• Introduction to music as a function of wavelength and harmonic number.

New Assignments:

• Sound bytes_ears are better than eyes
• speed of sound lab using tuning forks
• pop bottle physics

This week began with an transition from systems ‘fixed at both ends’ to systems ‘open at one end’ (sound waves in tubes) and an exploration of the harmonic sequence of wave forms. As part of this we used ‘variable length’ cylinder of air to determine the speed of sound (based on the resonance of sound waves for a given, fixed frequency tuning fork). And then considered the human voice (vocal chords, fixed at each end) and the myriad harmonics that give us our unique sounds..

• Gas Jets showing standing wave forms
• Polyphonic Overtone singer
• Blueman drumbeat
• Equal Tempered Frequencies
• Why its impossible to tune a piano

Learning Objectives

• Introduction to Pressure.
• Triple point of water.
• The formation of ‘shock waves’ as aircraft reach the speed of sound
• Buoyancy

New Assignments:

• Buoyancy lab. Students were given a wooden dowel and then tasked with first determining the density of the piece, then based on principals of buoyancy the students were to determine what fraction of the dowel would remain above water (note: meaning how many cm would remain above water). Students did this math individually including a drawing the system, all measured values and math along the way. (percent error too!). For Extra Credit, students also determined the uncertainty in the measurements and the percent uncertainty in their final value).
• (note: concrete canoes are a standard engineering project at university)

This week’s AP Test Practice. 

• FRQ: 1 + 2 from Unit 7: Oscillations. (students were to download the on-line Blue Book and use the secure web browser for this test today)

Introduction to pressure. Discussion was centered on fluids and the pressure that we experience when under water. The basic, introductory point was that the pressure we experience is nothing more than the weight of the water column at any given depth. But.. since fluids flow, the force of the weight of the water is not limited to just pointing down. Fluids want to ‘push out’ in all directions. (imagine a cylinder of water with a T-fitting at the bottom, clearly the weight of the water will push the water out sideways.).

A quick demonstration was done after bringing up the Digital Dutch webpage showing that 1 Atm. = 14.6 lbs/square inch. What does this mean? After filling up an Erlymeyer flask with water, and noting that the opening is roughly 2.5 square inches… means that the total force on the opening is roughly 38 lbs of force total. Clark then turned the flask upside down with nothing more than a piece of cardstock to close it up.

 

Learning Objectives

• Introduction to fluids in motion (fluid dynamics) and comparisons between Fluid Statics and Fluid Dynamics.
• Bernoulli’s Equations: 

New Assignments:

• (note: concrete canoes are a standard engineering project at university)

This week’s AP Test Practice. 

• FRQ: 1 + 2 from Unit 7: Oscillations. (students were to download the on-line Blue Book and use the secure web browser for this test today)

 

 

Learning Objectives

• Bernoulli’s equations (for fluid dynamics.. i.e., fluids in motion)

New Assignments:

• Chpt 11 problems: 5, 10, 11, 12, 25. (page 514 of text). (solutions to chpt 11 hw set (5, 10, 11, 12, 25)
• Video: Breaking the sound barrier. Students were to take a page of notes.
• Students took the AP Classroom, practice AP Test for Fluids today (both multiple choice and FRQ’s) 

Clark was out on Monday. Students worked on practice problems from chapter 11.

Video: Breaking the sound barrier. This Modern Marvels documentary describes the engineering challenges of trying to create a plane that could fly faster than sound and more importantly, be controllable. Chuck Yeager emerges as the hero of the day. Students were to take a page of notes.

Discussion: Bernoulli’s’ equations for fluids in motion. First we watched this video which is a great introduction to Bernoulli’s principals.

 

Learning Objectives

• Bernoulli’s equations (for fluid dynamics.. i.e., fluids in motion)

New Assignments:

• Chpt 11 problems: 5, 10, 11, 12, 25. (page 514 of text). (solutions to chpt 11 hw set (5, 10, 11, 12, 25)
• Video: Breaking the sound barrier. Students were to take a page of notes.
• Students took the AP Classroom, practice AP Test for Fluids today (both multiple choice and FRQ’s) 

Clark was out on Monday. Students worked on practice problems from chapter 11.

Video: Breaking the sound barrier. This Modern Marvels documentary describes the engineering challenges of trying to create a plane that could fly faster than sound and more importantly, be controllable. Chuck Yeager emerges as the hero of the day. Students were to take a page of notes.

Discussion: Bernoulli’s’ equations for fluids in motion. First we watched this video which is a great introduction to Bernoulli’s principals.